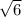Question

P is inversely proportional to the square of Q(P,Q>0). When P=3, Q=4. Find an equation for P in terms of Q and find the exact value of Q when P=8.

Answer 1

Q =
`√(6)`

Explanation:

given P is inversely proportional to Q² , then the equation relating them is

P =
`(k)/(Q^2)` ← k is the constant of proportionality

to find k , substitute P = 3 , Q = 4 into the equation

3 =
`(k)/(4^2)` =
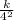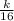`(k)/(16)` ( multiply both sides by 16 )

48 = k

P =
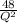`(48)/(Q^2)` ← equation of proportion

when P = 8 , then

8 =
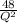`(48)/(Q^2)` ( multiply both sides by Q²

8 Q² = 48 ( divide both sides by 8 )

Q² = 6 ( take square root of both sides )

`√(Q^2)` = ±
`√(6)`

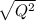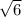
Since Q > 0 , then Q =
`√(6)`