Question

A sequence has the nth term rule

T(n) = 46 - 4n
What is the value of the first negative term in this sequence?

Answer 1

-2

Explanation:

The first negative term in the sequence is the first term that is less than zero. This means that we need to find the value of n for which T(n) < 0.

We can start by setting T(n) = 0 and solving for $n$:

46 - 4n = 0

-4n = -46

`\sf n =(-46)/(-4)`

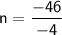
n = 11.5

Since ln must be an integer, the first negative term in the sequence is for n = 12.

To find the value of the first negative term, we can simply substitute n = 12 into the formula for T(n):

T(12) = 46 - 4(12)

T(12) = 46 - 48

T(12) = -2

Therefore, the value of the first negative term in the sequence is -2.