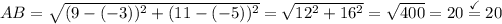Question

Plot the points A(9, 11) and B(–3, –5). Find midpoint M of AB. Then show that AM = MB and AM + MB =AB

Answer 1

The midpoint is (3, 3).

Explanation:

We are given the two points A(9, 11) and B(-3, -5).

The midpoint is given by:

`\displaystyle M=\Big((x_1+x_2)/(2),(y_1+y_2)/(2)\Big)`

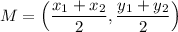
So:

`\displaystyle M = \Big( (9+(-3) )/(2), ( 11+(-5) )/(2) \Big) = (3,3)`

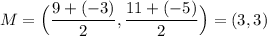
The midpoint is (3, 3).

We want to show that AM = MB.

We can use the distance formula:

`d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2`

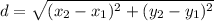
The distance between A(9, 11) and M(3, 3) will then be:

`AM=√((9-3)^2+(11-3)^2)=√(6^2+8^2)=√(100)=10`

And the distance between B(-3, -5) and M(3, 3) will be:

`MB = √( (3-(-3))^2 + (3-(-5))^2 ) = √((6)^2+(8)^2) = √( 100 ) = 10`

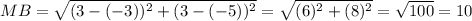
So, AM = MB = 10.

Since AM = MB = 10, AM + MB = 10 + 10 = 20.

So, we want to prove that AB = 20.

By the distance formula:

`AB=√((9-(-3))^2+(11-(-5))^2)=√(12^2+16^2)}=√(400)=20\stackrel{\checkmark}{=}20`