Question

3
x. 3x²+x-3=0
Find the zeros

Answer 1

The zeroes of this polynomial are
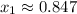`x_(1) \approx 0.847` and
`x_(2) \approx -1.180`.

Explanation:

Let
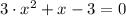`3\cdot x^(2)+x - 3 = 0`, the quickest and most efficient approach to find the zeroes of this second order polynomial is by Quadratic Formula. For all
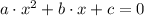`a\cdot x^(2)+b\cdot x + c = 0`, roots are determined by:

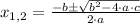`x_(1,2) = \frac{-b\pm \sqrt{b^(2)-4\cdot a\cdot c}}{2\cdot a}` (1)

Where
`a`,
`b`,
`c` are coefficients of the polynomial.

If we know that
`a = 3`,
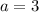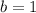`b = 1` and
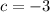`c = -3`, then roots of the polynomial are, respectively:

`x_(1,2) = \frac{-1\pm\sqrt{1^(2)-4\cdot (3)\cdot (-3)}}{2\cdot (3)}`

`x_(1,2) =(-1\pm √(37))/(6)`

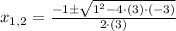
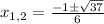
The zeroes of this polynomial are
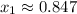`x_(1) \approx 0.847` and
`x_(2) \approx -1.180`.