Question

83. A sailboat travels south at 12.0 km/h with respect to the

water against a current 15.0° south of east at 4.0 km/h.
What is the boat's velocity?

Answer 1

The magnitude of the boat's velocity is 8.21 km/h.

Step-by-step explanation:

We can find the boat's velocity as follows:

`\Epsilon V_(x) = V_{w_(x)} + V_{b_(y)}`

`\Epsilon V_(y) = V_{w_(y)} + V_{b_(y)}`

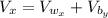
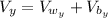
Where:

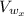`V_{w_(x)}` and
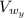`V_{w_(y)}` are the components of the velocity of the water in the x and y-direction

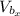`V_{b_(x)}` and
`V_{b_(y)}` are the components of the velocity of the boat in the x and y-direction

Since the angle is 15° we have:

`\Epsilon V_(x) = -4.0 km/h*sin(15) + 0 = -1.04 km/h`

`\Epsilon V_(y) = 4.0 km/h*cos(15) - 12.0 km/h = -8.14 km/h`

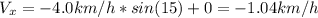
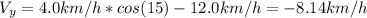
Now, the velocity of the boat is:

`V = \sqrt{V_(x)^(2) + V_(y)^(2)} = \sqrt{(-1.04 km/h)^(2) + (-8.14 km/h)^(2)} = 8.21 km/h`

Therefore, the magnitude of the boat's velocity is 8.21 km/h.

I hope it helps you!