Question

According to the rational root theorem, which of the following are possible

roots of the polynomial function below?
F(x) = 4x2 - 6x2 + 9x + 10

A.5/4
B.1/3
C.-1/2
D.5
E.6
F.-2

Answer 1

Given:

The polynomial function is

`F(x)=4x^3-6x^2+9x+10`

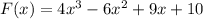
To find:

The possible roots of the given polynomial using rational root theorem.

Solution:

According to the rational root theorem, all the rational roots and in the form of
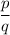`(p)/(q)`, where, p is a factor of constant and q is the factor of leading coefficient.

We have,

`F(x)=4x^3-6x^2+9x+10`

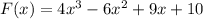
Here, the constant term is 10 and the leading coefficient is 4.

Factors of constant term 10 are ±1, ±2, ±5, ±10.

Factors of leading term 4 are ±1, ±2, ±4.

Using rational root theorem, the possible rational roots are

`x=\pm 1+,\pm 2, \pm 5, \pm 10,\pm (1)/(2), \pm (5)/(2), (1)/(4), \pm (5)/(4)`

Therefore, the correct options are A, C, D, F.