Question

Find the remaining sides of a 45°-45°-90° triangle if the longest side is 4. Answer exactly.

Answer 1

Answer:

Length of each leg= 2√2

Step by steps solved:

In a 45°-45°-90° triangle, the ratio of the sides is always 1 : 1 : √2.

If the longest side (hypotenuse) is 4, then the other two sides (legs) must be equal and can be found by dividing the hypotenuse by √2.

So, the length of each leg is:

4 / √2 = 4√2 / 2 = 2√2

Therefore, the length of each leg is 2√2.

Answer 2

each of the other sides is 2√2 = 2.8284

Explanation:

A 45°- 45°-90° triangle is also known as a right isosceles triangle because two of the legs are the same as two of the angles are the same at 45°

If a is the length of one of the legs then the hypotenuse, h, which is the longest side is given by the equation

`h = √(a^2 + a^2)\\\\h = √(2a^2)\\\\h = a √(2)`

Or, dividing by √2 on both sides,

`(h)/(√(2) )= a`

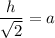
Given h = 4

`a =( 4)/(√(2)) = (2\cdot2)/(√(2)) = 2√(2) \quad\quad\quad(since $(2)/(√(2))$ = √(2)})`

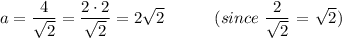
a = 2√2 = 2.8284

So each of the other sides is 2√2 = 2.8284