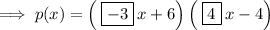Question

Enter values to write the function that matches the graph shown.

Answer 1

`p(x)=\left(\:\boxed{-3}\:x+6\right)\left(\:\boxed{4}\:x-4\right)`

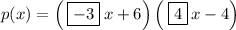
Explanation:

The x-intercepts are the points at which the curve crosses the x-axis.

From inspection of the given graph, the x-intercepts are:

• x = 1
• x = 2

As the parabola opens downwards, the leading coefficient is negative.

`\boxed{\begin{minipage}{6 cm}\underline{Intercept form of a quadratic equation}\\\\$y=a(x-r_1)(x-r_2)$\\\\where:\\ \phantom{ww}$\bullet$ $r_1$ and $r_2$ are the $x$-intercepts. \\ \phantom{ww}$\bullet$ $a$ is some constant.\\\end{minipage}}`

Therefore:

`p(x)=-a(x-1)(x-2)`

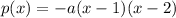
Expand:

`\implies p(x)=-a(x^2-3x+2)`

`\implies p(x)=-ax^2+3ax-2a`

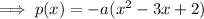
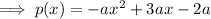
The y-intercept is the point at which the curve crosses the y-axis, so when x = 0. As the curve intersects the y-axis at y = -24:

`\begin{aligned}\implies p(0)=-a(0)^2+3a(0)-2a&=-24\\-2a&=-24\\a&=12\end{aligned}`

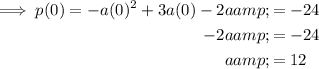
Therefore the equation in intercept form is:

`p(x)=-12(x-1)(x-2)`

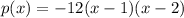
Rewrite -12 as 4 · -3:

`\implies p(x)=4 \cdot -3(x-1)(x-2)`

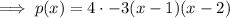
Multiply (x - 1) by 4 and (x - 2) by -3:

`\implies p(x)=4(x-1)(-3)(x-2)`

`\implies p(x)=(4x-4)(-3x+6)`

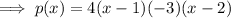
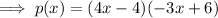
Applying the commutative property of multiplication:

`\implies p(x)=(-3x+6)(4x-4)`

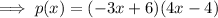
Compare with the given function:

`\implies p(x)=\left(\:\boxed{-3}\:x+6\right)\left(\:\boxed{4}\:x-4\right)`