Question

Given r(x) = StartFraction 11 Over (x minus 4) squared EndFraction , which represents a domain restriction on r(x) and the corresponding inverse function? X > 4; r–1(x) = 4 minus StartRoot StartFraction 11 Over x EndFraction EndRoot x > 4; r–1(x) = 4 + StartRoot StartFraction 11 Over x EndFraction EndRoot x > –4; r–1(x) = 4 minus StartRoot StartFraction 11 Over x EndFraction EndRoot x > –4; r–1(x) = 4 + StartRoot StartFraction 11 Over x EndFraction EndRoot

Answer 1

bbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbbb

Explanation:

b

Answer 2

x > 4; r–1(x) = 4 + StartRoot StartFraction 11 Over x EndFraction EndRoot

Explanation:

We are given that

`r(x)=(11)/((x-4)^2)`

We have to find the domain of restriction on r(x) and corresponding inverse function.

Let

`y=r(x)=(11)/((x-4)^2)`

`(x-4)^2=(11)/(y)`

`x-4=\sqrt{(11)/(y)}`

`x=\sqrt{(11)/(y)}+4`

`r^(-1)(y)=\sqrt{(11)/(y)}+4`

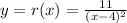
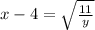
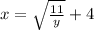
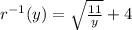
Now, replace x by y and y by x then, we get

`r^(-1)(x)=\sqrt{(11)/(x)}+4`

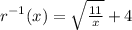
The function is not defined at x=4

But,

The inverse function defines for all positive real values.

Therefore, domain of r(x)=x>4 and inverse function

`r^(-1)(x)=\sqrt{(11)/(x)}+4`

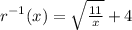
Option:

x > 4; r–1(x) = 4 + StartRoot StartFraction 11 Over x EndFraction EndRoot