Question

120 men working 9 hours a day can construct a road in 40 days.how long would it take to construct the same road if 150 men work 6 hours a day on it?

​

Answer 1

This is a question from Ratio and Proportion based concept.

Given that,

120 men working for 9 hours a day, construct a road in 40 days. We are required to calculate the number of days to construct the road if 150 people work for 6 hours.

Let's assume the number of days taken for constructing is 'x'.

So normally we take 2 ratios and obtain the unknown value by cross multiplication.

Applying the same approach we get:

`\implies ( 120\:men)/(150\:men)=(9\:hours)/(6\:hours)=(40\:days)/(x\:days)`

Since the first two fractions are inversely related, we multiply the numerators and denominators respectively.

Hence we get:

`\begin{gathered}\implies ( 120\: men * 9\: hours)/(150\: men * 6\: hours) = ( 40\: days)/(x\: days)\\\\\\\implies (1080)/(900) = (40)/(x)\\\\\\\sf{Cross~ multiplying ~these~ 2 ~fractions~ we~ get:}\\\\\\\implies 1080 * x = 40 * 900 \\\\\\\implies x = ( 40 * 900)/(1080)\\\\\\\implies x = (36000)/(1080)\\\\\\\implies x = \boxed{ 33.33 \:\: days}\end{gathered}`

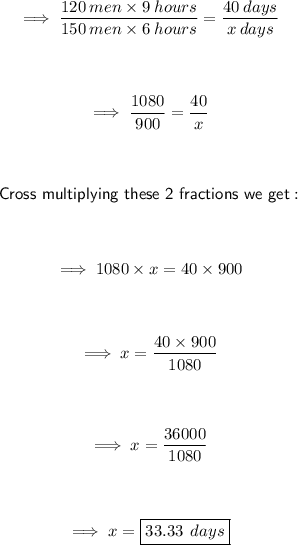
Hence it takes 33.33 days for 150 men to construct the road working for 6 hours each day.

Answer 2

Given :-

• 120 men working 9 hours a day can construct a road in 40 days.

To Find :-

• long would it take to construct the same road if 150 men work 6 hours a day on it?

Solution :-

Here,

• Let the time be y
• 120/150 = 9/6 = 40/y
• 120 × 9/150 × 6 = 40/y
• 1080/150 × 6 = 40/y
• 1080/900 = 40/y

By, Cross Multiplication

• 40 × 900 = 1080 × y
• 36,000 = 1080 × y
• 36,000 = 1080y
• 36,000/1080 = y
• 
  `\bold {y = 33.33 \: days}`

Therefore,

• 33.33 days for 150 men to construct the road working for 6 hours each day.