Question

What is an equation in point-slope form of the line that passes through (-7, 1) and (-3, 9)

Answer 1

y - y1 = m(x - x1) Is the answer

Answer 2

`{ \qquad\qquad\huge\underline{{\sf Answer}}}`

Here we go ~

First, calculate the slope of line joining points (-7 , 1) and (-3 , 9) :

`\qquad \sf  \dashrightarrow \: m = (y2 -y1 )/(x2 - x1)`

`\qquad \sf  \dashrightarrow \: m = (9 - 1)/( - 3 - ( - 7))`

`\qquad \sf  \dashrightarrow \: m = (8)/( - 3 + 7)`

`\qquad \sf  \dashrightarrow \: m = (8)/( 4)`

`\qquad \sf  \dashrightarrow \: m = 2`

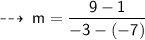
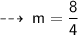
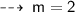
Slope of the line (m) = 2

Equation of line in point slope form :

`\qquad \sf  \dashrightarrow \: y - y1 = m(x - x1)`

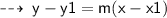
[ Put in the values ~ ]

`\qquad \sf  \dashrightarrow \: y - 9 = 2(x - ( - 3))`

`\qquad \sf  \dashrightarrow \: y - 9 = 2(x + 3)`

[ That's our required equation in point slope form ]

But it can be simplified further, it depends on it, if that's asked or not.

`\qquad \sf  \dashrightarrow \: y - 9 = 2x + 6`

`\qquad \sf  \dashrightarrow \: y = 2x + 6 + 9`

`\qquad \sf  \dashrightarrow \: y = 2x + 15`