Answer:
-4a²b
5a²b
Explanation:
A monomial is a polynomial that has one term only but can have multiple variables.
Given monomial:
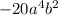
The coefficient of the given monomial is -20.
Therefore, we need to find two numbers that multiply to -20 and sum to 1.
Factors of -20:
- -1 and 20
- -2 and 10
- -4 and 5
- -5 and 4
- -10 and 2
- -20 and 1
Therefore, the two numbers that multiply to -20 and sum to 1 are:
Rewrite -20 as the product of -4 and 5:
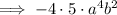
Rewrite the exponents as sums of equal numbers:
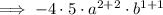

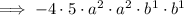
Rearrange as the product of two monomials with the same variables:
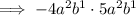
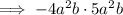
Therefore, the two monomials whose product equals -20a⁴b², and whose sum is a monomial with a coefficient of 1 are:
Check the sum of the two found monomials:
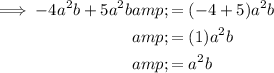
Thus proving that the sum of the monomials has a coefficient of 1.