Question

What is the sum of the infinite geometric series?.

1/2+1/4+1/8+1/16+...

Answer 1

`1/2+1/4+1/8+1/16+...=\sum\limits_(n=1)^\infty ((1)/(2))^n = ((1)/(2))/(1-(1)/(2))=1`

We can see that consecutive fractions are made from 1/2 to consecutive powers. Because we begin with 1/2, n=1
We will infinitely add fractions , hence Lemniscate sign.

In other words you cannot find a number which needs to be added to the geometric series to get "1", therefore the answer is 1. I remember the teacher explaining it this way :)

Answer 2

The sum of the given geometric series is, 1

Explanation:

Geometric sequence states that a sequence of numbers that follows a pattern were the next term is found by multiplying by a constant called the common ratio (r).

The sum of the infinite terms of a geometric series is given by:

`S_\infty = (a)/(1-r)` ......[1] ;where
`0<r<1`

Given the series:
`(1)/(2)+(1)/(4)+(1)/(8)+(1)/(16)+.....`

Since, this series is geometric series with constant term(r) =
`(1)/(2)`

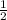
Since,

`((1)/(4))/((1)/(2) ) =(2)/(4) = (1)/(2)`,

`((1)/(8))/((1)/(4)) =(4)/(8) = (1)/(2)` and so on....

Here, first term(a) =
`(1)/(2)`

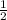
Substitute the values of a and r in [1] we get;

`S_\infty = ((1)/(2))/(1-(1)/(2))` where r =
`(1)/(2)< 1`

`S_\infty = ((1)/(2))/((2-1)/(2))`

or

`S_\infty = ((1)/(2))/((1)/(2))`

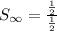
Simplify:

`S_\infty = 1`

Therefore, the sum of the infinite geometric series is, 1