Given the triangle ABC, you can identify that the coordinates of its vertices are:
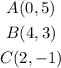
You know that it is reflected over the following line:
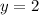
Notice that it has the form of a horizontal line. It intersects the y-axis at this point:

Knowing that you can graph the line. See the picture below:
By definition, when a figure is reflected over a line, the points of the Image (the figure obtained after the transformation) and the points of the Pre-Image (the original figure), have the same distance from the line of reflection.
Notice that:
- Point A is 3 units away from the line of reflection.
- Point B is 1 unit away from the line.
- Point C is 3 units away from the line.
See the picture attached:T
Therefore, the points of the Image will have this distance from the line of reflection:
- Point A' will be 3 units away from the line of reflection.
- Point B' will be 1 unit away from the line.
- Point C' will be 3 units away from the line.