We have been given a polynomial function
 . We are asked to choose the root of the function from given choices.
. We are asked to choose the root of the function from given choices.
Let us set our polynomial equal to 0.
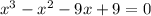
Now we will factor our polynomial by grouping method.
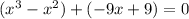
Let us factor out greatest common factor from each group.


We can further factor
 using difference of squares.
using difference of squares.

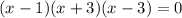
Using zero product property, we will get:
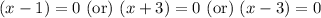
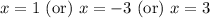
Upon looking at our given choices, we can see that
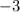 is the correct choice, therefore,
is the correct choice, therefore,
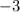 is root of the given polynomial.
is root of the given polynomial.