Question

The x-axis contains the base of an equilateral triangle RST. The origin is at S. Vertex T has coordinates (2h, 0) and the y-coordinate of R is g, with g > 0.

Enter the coordinates for the midpoint of ST:

Enter the x- coordinate of R:

Answer 1

Coordinates of Mid Point of ST is ( h , 0 ) and x-coordinate of R is
`\pm√(4h^2-g^2)`.

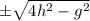Explanation:

Given: Coordinate of S ( 0 , 0 ) , Coordinate of T ( 2h , 0 )

y-coordinate of R = g

Coordinates of Mid point of ST =
`((2h+0)/(2),(0+0)/(2))`

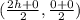
= ( h , 0 )

let x-coordinate of point R be x

Distance of RS = Distance of ST

`√((x-0)^2+(g-0)^2)=√((2h-0)^2+(0-0)^2)`

`√(x^2+g^2)=√((2h)^2)`

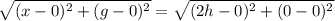
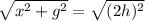
Squaring both sides, we get

`x^2+g^2=(2h)^2`

`x^2=(2h)^2-g^2`

`x=\pm√(4h^2-g^2)`

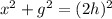
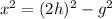
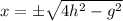
So, x-coordinate of R is
`\pm√(4h^2-g^2)`

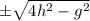
Therefore, Coordinates of Mid Point of ST is ( h , 0 ) and x-coordinate of R is
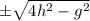`\pm√(4h^2-g^2)`.

Answer 2

For the first part remember that an equilateral triangle is a triangle in which all three sides are equal & all three internal angles are each 60°. So x-coordinate of R is in the middle of ST = (1/2)(2h-0) = h
And for the second since this is an equilateral triangle the x coordinate of point R is equal to the coordinate of the midpoint of ST, which you figured out in the previous answer. Hope this works for you