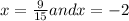Answer:
The factors of given quadratic equation
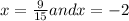
Explanation:
Explanation:-
Given quadratic equation 15x² +39 x -18 =0
The factors of 270 = 30 × 9
The quadratic equation 15x² +39 x -18 =0
⇒ 15 x² +30 x-9x -18=0
⇒ 15x(x+2) -9( x+2) =0
⇒( 15x -9)(x+2) = 0
15x - 9=0 and x+2 =0
15 x =9 and x = -2