Question

Derek wrote the following paragraph proof for the Vertical Angles Theorem:

The sum of angle 1 and angle 4 and the sum of angle 3 and angle 4 are each equal to 180 degrees by the definition of supplementary angles. The sum of angle 1 and angle 4 is equal to the sum of angle 3 and angle 4 by the transitive property of equality. Angle 1 is equal to angle 3 _____________________.

Which phrase completes the proof?

A. by construction using a straightedge
B. by the definition of a perpendicular bisector
C. by the subtraction property of equality
D. by the vertical angles theroem

Answer 1

Option C is correct.

By the Subtraction Property of Equality.

Step-by-step explanation:

Supplementary Angles: Two angles are Supplementary if the sum of the measure of angles is 180 degree.

Given:

`m\angle 1+ m\angle 4 =180^(\circ)`

`m\angle 3+ m\angle 4 =180^(\circ)` {By definition of supplementary angle} .......[1]

Transitive Property of equality:

If a=b and

b = c,

then a = c

By the transitive property,

[1} ⇒
`m\angle 1+m\angle 4 =m\angle 3+m\angle 4` ......[2]

Subtraction property of equality states that you subtract the same number from both sides of an equation.

Subtract
`m\angle 4` from both the sides in [2];

`m\angle 1+m\angle 4-m\angle4=m\angle 3+m\angle 4-m\angle 4`

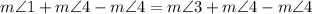
Simplify:

`m\angle 1 =m\angle 3` {By subtraction property of equality}

Therefore, the only phrase which completes the proof is; by the subtraction property of equality

Answer 2

the answer is C. by the subtraction property of equality
proof:
The sum of angle 1 and angle 4 is equal to the sum of angle 3 and angle 4 by the transitive property of equality
A1 + A4 = A3 + A4 so A1 = A3 + A4 - A4, and A1 = A3