Answer:
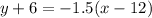 or
or
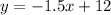 or
or

Explanation:
we know that
If two lines are perpendicular, then the product of their slopes is equal to minus one
so
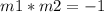
Step 1
In this problem we have
the given line
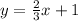
The slope of the given line is

Find the perpendicular slope m2
substitute in the formula
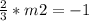
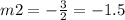
Step 2
Find the equation of the line
we have
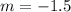
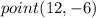
The equation of the line into point slope form is equal to

substitute
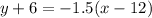 -------> equation of the line into point slope form
-------> equation of the line into point slope form
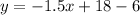
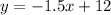 ------> equation of the line into slope intercept form
------> equation of the line into slope intercept form
The equation of the line in standard form is

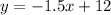
 -----> multiply by
-----> multiply by
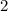
 -----> equation of the line in standard form
-----> equation of the line in standard form