Question

A round silo is 60 feet tall and has a 15 foot radius. about how high would a load of 32,000 ft³ of grain fill the silo to?

Answer 1

find volume

v=hpir^2
v=60pi15^2
v=60pi225
v=13500pi
v=42411.492=full

find wha fraction 32000 is
32000/42411.492=0.75451247
times that by height
60*0.75451247=45.2707487

about 45 feet

Answer 2

A load of 32000 cubic feet fill the silo to height 45.252 feet.

Explanation:

Height of silo = 60 feet

Radius of silo = 15 feet

Suppose silo in in the shape of cylinder

Volume of grain in silo =
`\pi r^2 h = (22)/(7) * 15^2 * h`

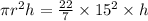
Volume of grain in silo =
`32000 ft^3`

`(22)/(7) * 15^2 * h =32000\\h=(32000 * 7)/(22 * 15^2)`

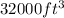
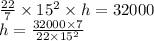
h=45.252 feet

Hence A load of 32000 cubic feet fill the silo to height 45.252 feet.