Question

two adjacent angles of a parallelogram are in the ratio 5:2: . find all the angles of the parallelogram​

Answer 1

Given :-

• Two adjacent angles of a parallelogram are in the ratio of 5 : 2.

To Find :-

• What is the all angles of the parallelogram.

Solution :-

Let, the first angles be 5x

And, the second angles will be 2x

As we know that,

★ Sum of all angles = 180° ★

According to the question by using the formula we get,

• ⇒ 5x+2x=180°

• ⇒7x=180°

• ⇒
  `\sf x =\: \frac{\cancel{180^(\circ)}}{\cancel{7}}`

• ➠
  `\sf\bold{\red{x =\: 25.7^(\circ)}}`

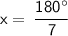
Hence, the required angles are,

• First angles = 5x = 5(25.7°) = 128.5°
• Second angles = 2(25.7°) = 51.4°

As we know that,

opposite angles in a parallelogram

is equal.

∴ The all angles of a parallelogram is 128.5°,51.4°, 128.5° and 51.4°.