Question

What is the length (in cm) of the side of an iron ingot (rectangular box) that has a mass of 4.578 kg and has width = height = 5.00 cm

Show calculations please

Answer 1

since we have the mass of the iron ingot we can get its volume by dividing the mass (4.578) by the density of iron (7874 kgm^-3) and we get that the volume is 5.8140716x10^-4 m^3. now we convert the volume from m^3 to cm^3(1m^3=1000000cm^3) and so by multiplying 5.8140716x10^-4 by 1000000 we get:581.4cm^3 (1 decimal place).

the volume for any cuboid is V=l x w x s we have the volume, the length and the width, so we plug in the values and solve for s

`s= (581.4)/(5^(2) ) =23.3cm`
the answer is rounded to three significant figures

hope i helped

Answer 2

Answer : The length of the side of an iron ingot (rectangular box) is 0.0233 cm.

Explanation : Given,

Width of rectangular box = 5.00 cm

Height of rectangular box = 5.00 cm

Mass of rectangular box = 4.578 g

Density of iron =
`7.86cm^3`

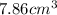
First we have to determine the volume of rectangular box.

Formula used :

`\text{Density}=\frac{\text{Mass}}{\text{Volume}}`

`7.86cm^3=\frac{4.578g}{\text{Volume}}`

`\text{Volume}=0.582cm^3`

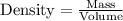
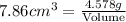
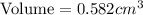
Now we have to determine the length of rectangular box.

Formula for volume of rectangle :

`V=l* b* h`

where,

V = volume of rectangular box

l = length of rectangular box

b = width of rectangular box

h = height of rectangular box

Now put all the given values in the above formula, we get:

`0.582cm^3=l* (5.00cm)* (5.00cm)`

`l=0.0233cm`

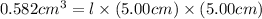
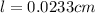
Therefore, the length of the side of an iron ingot (rectangular box) is 0.0233 cm.