Answer:
(B). The new volume of the cylinder is
 of the original volume.
of the original volume.
Step-by-step explanation:
Let 5 r be the radius and h be the height of the cylinder.
The volume of the cylinder is

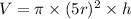

A cylinder’s radius is reduced to 2/5 its original size and the height is quadrupled.
The new radius of the cylinder is
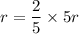
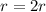
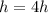
The new volume of the cylinder is
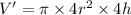
The ratio of the original volume and the new volume
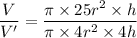
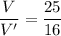
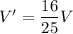
Hence, The new volume of the cylinder is
 of the original volume.
of the original volume.