Question

What is the area of the two rectangles shown below?

Answer 1

-- I don't know if you'll still need mine, but here's what I got --

1st (shaded) box = Area is 441

2nd (shaded) box = Area is 1,764

Explanation:

First of all, It stated, "To determine the area of the (shaded) portion" but it didn't mention the white box so I ignored it and pay attention only to the "shaded box" as mentioned in the assignment

1st (shaded) box

1. write it out like so,
`4x + 7 = 3x`

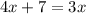
2. next to get "x" alone subtract 7 to the other side once done you would have this,
`4x=3x-7`

3. then subtract 3x to both sides...
`3x-3x=cancelsout` >>
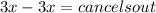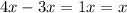`4x-3x=1x=x` >> you're now left with,
`x=-7`

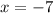
To find the area we need one more step...

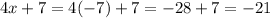`4x+7=4(-7)+7=-28+7=-21` and
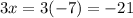`3x=3(-7)=-21` then multiply to get the area, negative crosses each other out once multiplying so -21 times -21 gives you 441

-------

2nd (shaded) box repeat from above

1.
`8n+14=6n`

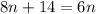
2.
`8n=6n-14`

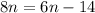
3.
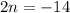`2n=-14`, here is different as there is still one more step, we need to divide to get "n" alone.

4.
`(2n=-14)/(2) = n=-7`

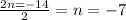
Now plugin to get the area,
`6n=6(-7)=-42\\8n+14=8(-7)+14=-56+14=-42\\` once multiplied you get 1,764

Once again I could be wrong but this is what I got after reading the little description from your attached assignment.

Answer 2

Answers are in bold.

For the first one:

The total area of both shaded and unshaded portions is equal to
`3x(4x+7)`, which simplifies to
`12x^2}+21x`. The area of the middle white portion is
`(5x)(2x)`, which simplifies to
`10x^2`. To find the area of the shaded portion, we do
`12x^2}+21x - 10x^2`. Combining like terms, we get
`2x^2+21x` as our expression for the area of the shaded portion of the first rectangle.

For the second one:

The total area of both shaded and unshaded portions is equal to
`6n(8n+14)`, which simplifies to
`48n^2}+84n`. The area of the middle white portion is
`10n(4n+1)`, which simplifies to
`40n^2+10n`. To find the area of the shaded portion, we do
`48n^2+84n-40n^2+10n`. Combining like terms, we get
`4n^2+94n` as our expression for the area of the shaded portion of the second rectangle.