Question

Justify why a/b x b/c x c/d x d/ e is equal to a/e when b,c,d, and e are not zero?

Answer 1

`(a)/(b) * (b)/(c) * (c)/(d) * (d)/(e)= (a)/(e)`

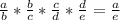
lets rewrite the equation so as to better understand why this equation is true..


`(a)/(b) * (b)/(c) * (c)/(d) * (d)/(e)= (abcd)/(bcde)= (a)/(e) * (b)/(b) * (c)/(c) * (d)/(d)`
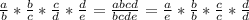
If both the numerator and the denominator are the same in a fraction, then that fraction is equivalent to 1. So we can rewrite the equation as such.


`(a)/(e) * 1 * 1 *1= (a)/(e)`

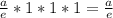
Since the b's, c's and d's cancel out, a/b*b/c*c/d*d/e=a/e