Question

Given the function f(x) = −3x3 + 9x2 − 2x + 3, what part of the function indicates that the left end starts at the top of the graph?

The degree of the first term

The degree of the last term

The coefficient of the first term

The coefficient of the last term

Answer 1

The coefficient and degree of the first term .

Explanation:

Given :f( x) =
`-3x^(3) +9x^(2) -2x+3`.

To find : what part of the function indicates that the left end starts at the top of the graph.

Solution : We have given that f( x) =
`-3x^(3) +9x^(2) -2x+3`.

End behavior of end point of a function : The degree and the leading coefficient of a polynomial function determine the end behavior of the graph.

Therefore, The coefficient and degree of the first term .

Answer 2

Answer: The correct option is, The coefficient of the first term.

Explanation:

The given function is,

`f(x)=-3x^3+9x^2-2x+3`

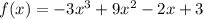
End behavior of the polynomial function : It is defined as the graph of f(x) as x approaches
`+\infty` and
`-\infty`.

The end behavior of the graph depends on the leading coefficient and degree of the polynomial.

As, the degree of the polynomial is '3'. So, the leading coefficient will determine the structure of the graph.

Therefore, the coefficient of the first term will indicate that the left end starts at the top of the graph.

The graph is also shown below.