Answer: 4030
Explanation:
We know that sum of an arithmetic series with first term as 'a' and the last term as 'l' is given by :-
 , where n is the number of terms
, where n is the number of terms
In the given situation, the number of terms = 52
The first term = 1
The 52th term = 154
Then the sum of 52 terms ids given by :-
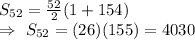
Hence, the sum of the series =4030