Question

Quadrilateral ABCD is located at A(-2,2) B(-2,4) G(2,4) D(2,2). The quadrilateral is then transformed using the rule (x+2,y-3) to form the image A'B'C'D'. What are the new coordinates of of A'B'C'D'? Describe what characteristics you would find if the corresponding vertices were connected with line segments.

Answer 1

Answer: New coordinates are

A'(0,-1), B' (0,1) , C' (4,1) and D' (4,-1)

And A'B'C'D' is rectangle.

Explanation:

Since we have given that

Quadrilateral ABCD is located at

A (-2,2)

B (-2,4)

C (2,4)

D (2,2).

Using the rule (x+2,y-3), we need to form the image A'B'C'D'.

New Coordinates of A'B'C'D' will be

`A=(-2,2)\implies A'=(-2+2,2-3)=(0,-1)\\\\B=(-2,4)\implies B'=(-2+2,4-3)=(0,1)\\\\C=(2,4)\implies C'=(2+2,4-3)=(4,1)\\\\D=(2,2)\implies D'=(2+2,2-3)=(4,-1)`

So, we can see that A'B'C'D' is rectangle as opposite sides are equal in length and each angle is right angle as shown in the figure below.

Answer 2

A'(0,1),B'(0,-1), C'(4,1), D'(4,-1)

Rectangle

Explanation:

We are given that a quadrilateral ABCD is located at A (-2,2), B(-2,4), C(2,4) and D (2,2).

We have to find the new coordinates of A'B'C'D' after translation by the rule (x+2,y-3) of quadrilateral ABCD.

Apply the given rule of transformation

`(x, y)\rightarrow (x+2,y-3)`

`A=(-2,2)\rightarrow (0,-1)=A'`

`B=(-2,4)\rightarrow (0,1)=B'`

`C=(2,4)\rightarrow (4,1)=C'`

`D=(2,2)\rightarrow (4,-1)=D'`

`A'B'=√((0-0)^2+(1+1)^2)=2 units`

`B'C'=√((4)^2+(1-1)^2)=4 units`

`C'D'=√((4-4)^2+(-1-1)^2)=2 units`

`A'D'=√((4)^2+(-1+1)^2)=4 units`

`A'B'=C'D', B'C'=A'D'`

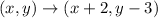
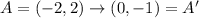
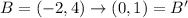
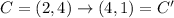
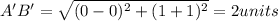
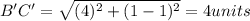
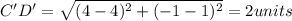
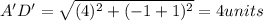
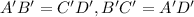
When we meet the vertices with the line segments then the shape formed is rectangle because opposite sides are equal and each angle is equal to 90 degrees in given figure.