Question

Find the product of (3x − 7y)^2.

Answer 1

(3x - 7y)^2 =
(3x - 7y)(3x - 7y) =
3x(3x - 7y) - 7y(3x - 7y) = 9x^2 - 21xy - 21xy + 49y^2...now combine like terms = 9x^2 - 42xy + 49y^2

Answer 2

The product of given expression
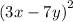`\left(3x-7y\right)^2` is
`9x^2-42xy+49y^2`

Explanation:

Given : The expression
`\left(3x-7y\right)^2`

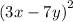
We have to find the product of the given expression
`\left(3x-7y\right)^2`

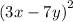
Apply perfect square formula ,
`\quad \left(a-b\right)^2=a^2-2ab+b^2`

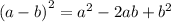
we have a = 3x and b = 7y

we get,

`=\left(3x\right)^2-2\cdot \:3x\cdot \:7y+\left(7y\right)^2`

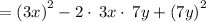
Simplify, we have,

`=9x^2-42xy+49y^2`

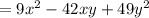
Thus, The product of given expression
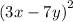`\left(3x-7y\right)^2` is
`9x^2-42xy+49y^2`