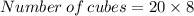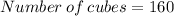Answer:
The number of cubes required is
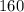 .
.
Explanation:
The dimensions of the right rectangular prisms are
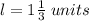
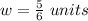
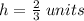
The volume of the right rectangular prism is
 .
.
We substitute the dimensions to get,
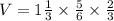 .
.
We convert the first mixed number to improper fraction,
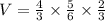 .
.
We multiply out to obtain,
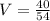
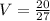 cubic units.
cubic units.
We need to determine the volume of the cube of side length,
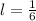 units.
units.
The volume of a cube is given by,

This implies that,
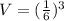
This gives us,
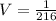 cubic units.
cubic units.
We now divide the volume of the right rectangular prism by the volume of the cube to determine the number of cubes required.
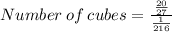
We simplify to get,
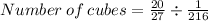
This implies that,