Question

1.Which is an equation of a circle with center (-5, -7) and radius 6?

2.Which is an equation of a circle with center (5, 0) that passes through the point (1, 1)?

3.What is the center and radius of the circle with equation (x - 5)2 + (y + 3)2 = 16?

4.Which point is on the circle described by (x - 2)2 + (y + 3)2 = 4?

Answer 1

1.
`(x+5)^2+(y+7)^2=36`

2.
`(x-5)^2+y^2=17`

3.

`r=4\\\\(h,k)=(5,-3)`

4.

`(2,-1)\\\\and\\\\(2,-5)`

Explanation:

A circle is the set of all points in a plane at a given distance (radius) from a given point (center). The standard equation of a circle with center (h,k) and radius r is given by:

`(x-h)^2+(y-k)^2=r^2`

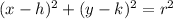
Therefore, we just need to use the previous equation in order to solve the problems.

1. Replacing the data provided into the equation of the circle:

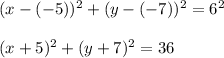
`(x-(-5))^2+(y-(-7))^2=6^2\\\\(x+5)^2+(y+7)^2=36`

2.

`(h,k)=(5,0)\\(x_o,y_o)=(1,1)`

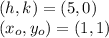
In this case, we have the center of the circle and a point of the circle, so, let's use that information in order to find the radius:

`(1-5)^2+(1-0)^2=r^2\\\\(-4)^2+(1)^2=r^2\\\\17=r^2\\\\r=√(17)`

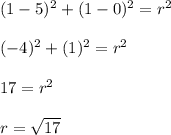
Hence, the equation of a circle with center (5, 0) that passes through the point (1, 1) is:

`(x-5)^2+(y-0)^2=(√(17) )^2\\\\(x-5)^2+y^2=17`

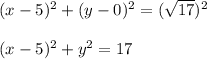
3. We can extract the solution directly from the equation:

`(x-5)^2+(y+3)^2=16`

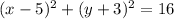
The radius is:

`r^2=16\\\\r=√(16) \\\\r=4`

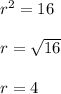
And the center is:

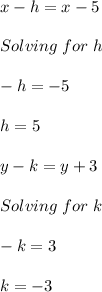
`x-h=x-5\\\\Solving\hspace{3}for\hspace{3}h\\\\-h=-5\\\\h=5\\\\y-k=y+3\\\\Solving\hspace{3}for\hspace{3}k\\\\-k=3\\\\k=-3`

So:

`Center=(h,k)=(5,-3)`

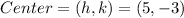
4. We can find one of the points on this circle:

`(x-2)^2+(y+3)^2=4`

Simply, eliminating one of the variables. For example, let's elimate x evaluating the equation for x=2:

`(2-2)^2+(y+3)^2=4\\\\(0)^2+y^2+6y+9=4\\\\y^2+6y+5=0`

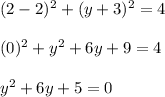
Solving for y:

`(y+5)(y+1)=0`

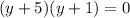
Hence:

`y=-1\\\\or\\\\y=-5`

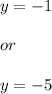
So, two points that are on the circle are:

`(2,-1)\\\\and\\\\(2,-5)`

Let's verify it evaluating the points into the equation of this circle.

For (2, -1)

`(2-2)^2+(-1+3)^2=4\\\\0+(2)^2=4\\\\4=4`

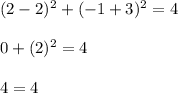
And for (2, -5)

`(2-2)^2+(-5+3)^2=4\\\\0+(-2)^2=4\\\\4=4`

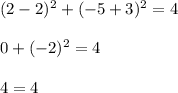
Since they satisfy the equation we can conclude that those points are on the circle.

Additionally I leave you the graph of every circle.

Answer 2

1. Equation of circle = (x - a)^2 + (y - b)^2 = r^2; where (a, b) is the centre of the circle and r is the diameter.
(x - (-5))^2 + (y - (-7))^2 = 6^2
(x + 5)^2 + (y + 7)^2 = 36
x^2 + 10x + 25 + y^2 + 14y + 49 = 36
x^2 + y^2 + 10x + 14y + 38 = 0