Question

Jake has proved that a function, f(x), is a geometric sequence. How did he prove that?. A) He showed that an explicit formula could be created. B) He showed that a recursive formula could be created. C) He showed that f(n) ÷ f(n - 1) was a constant ratio. D) He showed that f(n) - f(n - 1) was a constant difference.

Answer 1

The correct answer to this question is letter "C. He showed that f(n) ÷ f(n - 1) was a constant ratio." Jake has proved that a function, f(x), is a geometric sequence. He proves that he showed that f(n) ÷ f(n - 1) was a constant ratio.

Answer 2

Answer: The correct option is C) He showed that f(n) ÷ f(n - 1) was a constant ratio.

Step-by-step explanation: Given that Jake has proved that a function f(x) is a geometric sequence.

We are to select the correct method that he used in the proof.

GEOMETRIC SEQUENCE: A geometric sequence is a sequence of numbers where each term is found by multiplying the preceding term by a constant called the common ratio, r.

So, in Jame's proof, he showed that each term is multiplied by a constant to get the next term.

That is, if 'c' is the constant that was used in the proof, then we must have

`f(n)=c* f(n-1),\textup{ where 'n' is a natural number}>1.`

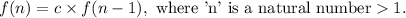
This implies that

`(f(n))/(f(n-1))=c\\\\\Rightarrow f(n)/ f(n-1)=c.`

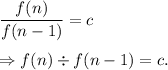
Therefore, he showed that f(n) ÷ f(n - 1) was a constant ratio.

Thus, (C) is the correct option.