Answer:
Option 'A' is true.
Explanation:
Given the equation
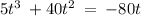
Add 80 to both sides
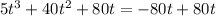
Simplify
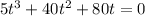
as 5t³ + 40t² + 80t = 5t (t + 4)²
5t (t + 4)² = 0
Using the zero factor principle
if ab=0, then a=0 or b=0 (or both a=0 and b=0)
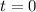 or
or
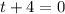
solving
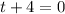
subtract 4 from both sides
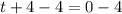
Simplify
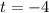
Therefore, the solution to the equation is:
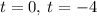
Hence, option 'A' is true.