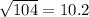Question

Assume that automobile accidents at a dangerous intersection occur according to a Poisson process at the rate of 3 accidents per week. Furthermore, the num- ber of people seriously injured in an accident has a Poisson distribution with mean 2 and is independent of the other accidents. Find the mean and standard deviation of the number of serious injuries over a one year's time.

Answer 1

The mean number of serious injuries over a one year's time is of 104 and the standard deviation is of 10.2

Explanation:

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given interval. The standard deviation is the square root of the mean.

Find the mean and standard deviation of the number of serious injuries over a one year's time.

Per week, mean
`\mu = 2`

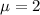
A year has approximately 52 weeks, so, the mean is
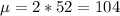`\mu = 2*52 = 104`, and the standard deviation is
`√(104) = 10.2`