Question

After landing on an unfamiliar planet, a space explorer constructs a simple pendulum of length 47.0 cm . The explorer finds that the pendulum completes 91.0 full swing cycles in a time of 125 s. What is the magnitude of the gravitational acceleration on this planet?

Answer 1

`g=9.83\ m/s^2`

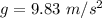
Step-by-step explanation:

Given that,

The length of a simple pendulum, l = 47 cm = 0.47 m

The pendulum completes 91.0 full swing cycles in a time of 125 s.

The time period of a simple pendulum is given by :

`T=2\pi \sqrt{(L)/(g)} \\\\or\\\\g=(4\pi^2L)/(T^2)`

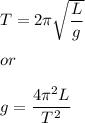
Also,

`T=(1)/(f)\\\\T=(t)/(n)`

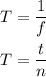
t is 125 s and n = 91 cycles

Substitute all the values to find g.

`g=(4\pi^2* 0.47)/(((125)/(91))^2)\\\\g=9.83\ m/s^2`

So, the gravitational acceleration on this planet is
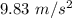`9.83\ m/s^2`.