Answer:
Booth workers required = 4
Explanation:
As given ,
Arrival rate = λ = 60 customers per hour
Time taken = 3.75 minutes
∴ we get
Average service rate = μ =
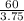 = 16 customers per hour
= 16 customers per hour
Now,
Minimum numbers of booth workers required =
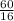 = 3.75
= 3.75
As workers can not be in fraction
∴ Minimum number of booth workers required = 4