Question

Find the lowest common denominator of p+3/p^2+7 p+10 and p+5/p^2+5 p+6

Answer 1

The answer you are looking for is (p+3)(p+2)(p+5).

Answer 2

We first need to factorize (if possible) the denominators:

we can see that
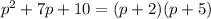
`p^2+7p+10=(p+2)(p+5)` as 2 and 5 are two numbers whose sum is 7 and product is 10.


Similarly, we can see that
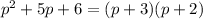
`p^2+5p+6=(p+3)(p+2)` as 2 and 3 are two numbers whose sum is 5 and product is 6.

Thus, the expression is:


`\displaystyle{ (p+3)/((p+2)(p+5)) + (p+5)/((p+3)(p+2))`.


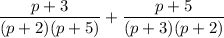
Now to make the denominators equal, but to also keep them as small as possible, the common denominator must be (p+3)(p+2)(p+5).


Answer: (p+3)(p+2)(p+5).