Question

A polynomial function has a root of 0 with multiplicity 1, and a root of 2 with multiplicity 4. If the function has a negative leading coefficient, and is of odd degree, which of the following are true? A. The function is positive on (-infinity, 0)

B. the function is negative on (0, 2) C. the function is negative on (2, infinity) D. the function is positive on (o, infinity)

Answer 1

A,B,C

Explanation:

I jus got it right on edge.

Answer 2

Answer: A,B and C are true.

Explanation:

let f(x) be the given polynomial with variable x such that

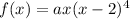`f(x)=ax(x-2)^4`m,where a be any odd degree negative leading coefficient of f(x),x has root as 0 with multiplicity 1 and
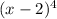`(x-2)^4` has root 2 with multiplicity 4.

Lets check all the options

A. The function is positive on (-∞, 0)

let x=-1∈(-∞, 0)

⇒
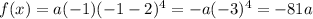`f(x)=a(-1)(-1-2)^4=-a(-3)^4=-81a`> 0 as a is negative.

∴ function is positive on (-∞, 0) .i,e. A is true.

B. The function is negative on (0, 2).

Let x=1 ∈(0,2)

⇒
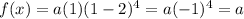`f(x)=a(1)(1-2)^4=a(-1)^4=a`< 0 as a is negative.

∴ the function is negative on (0, 2) .i,e. B is true.

C. The function is negative on (2, ∞)

let x=3∈(2,∞)

⇒
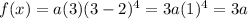`f(x)=a(3)(3-2)^4=3a(1)^4=3a`< 0 as a is negative.

∴ the function is negative on (2,∞).

D.The function is positive on (0, ∞) which is not true from C.