Solution:
Given;
A data set of values has a mean of 45 and standard deviation of 5.
The z-score for a point A is 0. The z-score for a point B is 0.2.
To find the values of A and B, we will apply the z-score formula, which is

Where
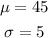
For the value of point A,
Where, z = 0 for point A
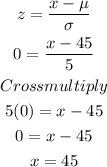
Hence, the value of point A is 45
For the value of point B,
Where, z = 0.2 for point B
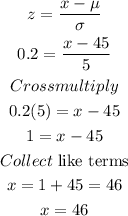
Hence, the value of point B is 46