2 Answers

Teilmann · Answer 1 · 2023-03-14T00:16:31+0000

Answer:

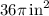
$36\pi \, \textrm{in}^2$

Explanation:

The surface area of a cylinder is defined as the surface area of the side + the surface area of its bases, which are circles.

First, find the surface area of the bases using the formula:

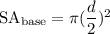
$\textrm{SA}_{\textrm{base}} = \pi ((d)/(2))^2$

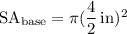
$\textrm{SA}_{\textrm{base}} = \pi ((4)/(2) \, \textrm{in})^2$

$\textrm{SA}_{\textrm{base}} = 4\pi \, \textrm{in}^2$

Next, find the surface area of the sides, which is the circumference of the bases multiplied by the height of the cylinder:

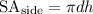
$\textrm{SA}_{\textrm{side}} = \pi d h$

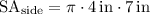
$\textrm{SA}_{\textrm{side}} = \pi \cdot 4 \, \textrm{in} \cdot 7 \, \textrm{in}$

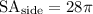
$\textrm{SA}_{\textrm{side}} = 28\pi$

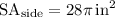
$\textrm{SA}_{\textrm{side}} = 28\pi \, \textrm{in}^2$

Finally, to find the surface area of the entire cylinder, add the area of the bases and the area of the side together (remember there are 2 bases):

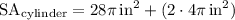
$\textrm{SA}_{\textrm{cylinder}} = 28\pi \, \textrm{in}^2 + (2 \cdot 4\pi \, \textrm{in}^2)$

$\textrm{SA}_{\textrm{cylinder}} = 28\pi \, \textrm{in}^2 + 8\pi \, \textrm{in}^2$

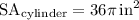
$\textrm{SA}_{\textrm{cylinder}} = 36\pi \, \textrm{in}^2$

So, the candle has a surface area of
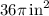$36\pi \, \textrm{in}^2$ .

Your comment on this question: