Question

Please help me understand how to...

Calculate the mass of Cr2O3 that can be produced if the reaction of 56.2 g of chromium and sufficient oxygen has a 76.0 % yield.
...Thank you!

Answer 1

`m_(Cr_2O_3)^(actual)=62.4gCr_2O_3`

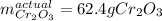
Step-by-step explanation:

Hello!

In this case, according to the reaction:

`4Cr+3O_2\rightarrow 2Cr_2O_3`

We can see there is a 4:2 mole ratio between chromium and chromium (III) oxide, this, for the given 56.2 g of chromium, the theoretical yield of the oxide product is computed down below:

`m_(Cr_2O_3)^(theoretical)=56.2gCr*(1molCr)/(52.0gCr)*(2molCr_2O_3)/(4molCr) *(151.99gCr_2O_3)/(1molCr_2O_3) =82.13gCr_2O_3`

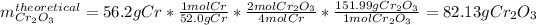
Now, considering the 76.0-% yield for this reaction, the actual yield turns out:

`m_(Cr_2O_3)^(actual)=82.13gCr_2O_3*(76.0gCr_2O_3)/(100gCr_2O_3) \\\\m_(Cr_2O_3)^(actual)=62.4gCr_2O_3`

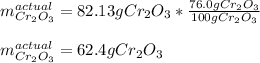
Best regards!