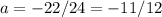Answer:
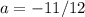
Explanation:
We are given the function:
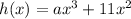
And we want to determine a such that h(x) has an inflection point at x = 4.
Possible inflection points are whenever h''(x) equals 0. So, we will first differentiate h(x) twice. This yields:
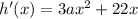
So:

Inflection points occur when h''(x) = 0. So:
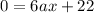
Since we have an inflection at x = 4:
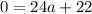
And solving for a yields: