Assuming you know the asymptote rules. We want to use the one that states if numerator's degree = 1 + denominators degree, the asymptote is a slant symptote in the form of y = mx + b.
![\lim_(n \to \infty) (f(x))/(x) \ \textgreater \ \lim_(n \to \infty) [tex] \lim_(n \to \infty) ( (x + 9)/(x^2) + 2x + 3 - 2x) \ \textgreater \ refine \ \textgreater \ \lim_(n \to \infty) ( (x + 9)/(x^2) + 3)](https://img.qammunity.org/2018/formulas/mathematics/college/znz3babaq7uas1r3m4wgnljha1brisigc7.png)
\ \textgreater \ 2 [/tex]
Now write the exception of indeterminate form.
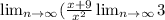
For
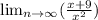
we want to divide by the highest denominator power.
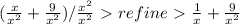
Write the exception again.
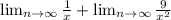
Apply the infinite property to both!

For 3 apply
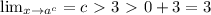
Now combine our terms and we get y = 2x + 3