Question

Which expression represents

the area of a rectangle with
sides measuring 7x4y2z units and
2xy5z14?

Answer 1

`\boxed{\boxed{\sf~a:14x^5y^7z^(15)}}`

Solution Steps:

______________________________

1.) To solve this you must first know the formula for area of a rectangle:

• Area
  `=\sf{H}` ×
  `\sf{W}`

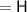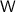
• Area
  `=7x^4y^2z` ×
  `2xy^5z^(14)`

Note:

- It doesn't matter which measurement fills the height or width part in the formula.

Equation at the end of Step 1:

• 
  `7x^4y^2z` ×
  `2xy^5z^(14)`

• 
  `2xy^5z^(14)` ×
  `7x^4y^2z`

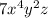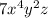
2.) Add 4 and 1, (x powers:)

• 
  `x^4+x=x^5`

Note:

- To multiply powers of the same base, you can add their exponents. So you just do
`4+1=5`.

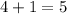- When you have a plain variable like
`x`, you can assume
`x=1`.

Equation at the end of Step 2:

• 
  `7x^5y^2z` ×
  `2y^5z^(14)`

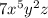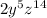
3.) Add 2 and 5, (y powers:)

• 
  `y^2+y^5=y^7`

Note:

- To multiply powers of the same base, you can add their exponents. So you just do
`2+5=7`.

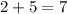Equation at the end of Step 3:

• 
  `7x^5y^7z` ×
  `2z^(14)`

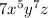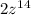
4.) Add 1 and 14, (z powers:)

• 
  `z+z^(14)=z^(15)`

Note:

- To multiply powers of the same base, you can add their exponents. So you just do
`1+14=15`.

- When you have a plain variable like
`z`, you can assume
`z=1`.

Equation at the end of Step 4:

• 
  `7x^5y^7z^(15)` ×
  `2`

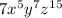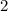
5.) Multiply 7 and 2:

• 
  `7` ×
  `2=14`

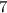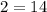
Note:

- Since we combine all the powers and multiplied the bases, all you have to do is put it all together in 1 form like we were doing after we added powers in the earlier steps.

Equation at the end of All Steps:

• 
  `14x^5y^7z^(15)`

______________________________