Part A
The probability of making a type ii error is equal to 1 minus the power of a hypothesis testing.
The power of a hypothesis test is given by:
![\beta(\mu')=\phi\left[z_(\alpha/2)+ (\mu-\mu')/(\sigma/√(n)) \right]-\phi\left[-z_(\alpha/2)+ (\mu-\mu')/(\sigma/√(n)) \right]](https://img.qammunity.org/2018/formulas/mathematics/college/ry0v5hhll83es7x0otgxv7nw2i918sumug.png)
Given that the machine is overfilling by .5 ounces, then
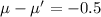
, also, we are given that the sample size is 30 and the population standard deviation is = 0.8 and α = 0.05
Thus,
![\beta(16.5)=\phi\left[z_(0.025)+ (-0.5)/(0.8/√(30)) \right]-\phi\left[-z_(0.025)+ (-0.5)/(0.8/√(30)) \right] \\ \\ =\phi\left[1.96+ (-0.5)/(0.1461) \right]-\phi\left[-1.96+ (-0.5)/(0.1461) \right] \\ \\ =\phi(1.96-3.4233)-\phi(-1.96-3.4233) \\ \\ =\phi(-1.4633)-\phi(-5.3833)=0.07169](https://img.qammunity.org/2018/formulas/mathematics/college/632oon4ke1ner3brxup6jsti3i67sr85dg.png)
Therefore, the probability of making a type II error when the machine is overfilling by .5 ounces is 1 - 0.07169 = 0.9283
Part B:
From part A, the power of the statistical test when the machine is overfilling by .5 ounces is 0.0717.