Question

Which of the following equations are true? Select three that apply.

A (2x−1) (x2+4x+3)= 2x3+7x2+2x−3
B (4x2−3x+6)+(5x2−8)=9x2−3x−2
C (8x−4x2)+(2x2−5x)=−2x4+3x
D (4x2+4x−5)−(6x2−5x−3)=−2x2−x−8
E (3x3−5+2x)−(5x−6+x3)=2x3−3x+1

Answer 1

`\boxed{\boxed{\sf~a,b,and~e~are~True.}}`

Solution Steps:

______________________________

a.)
`\bold{(2x-1) (x^2+4x+3)= 2x^3+7x^2+2x-3}`:

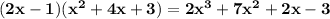Use the distributive property to multiply
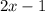`2x-1` by
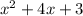`x^2+4x+3` and combine like terms:

`=2x^3+7x^2+2x-3`

- So this is True.

b.)
`\bold{(4x^2-3x+6)+(5x^2-8)=9x^2-3x-2}`:

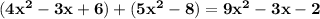Combine like terms and subtract 8 from 6 to get −2:

`=9x^2-3x-2`

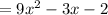
- So this is True.

c.)
`\bold{(8x-4x^2)+(2x^2-5x)=-2x^4+3x}`:

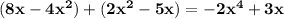Combine like terms:

`=3x-2x^2`

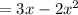
- So this is False.

d.)
`\bold{(4x^2+4x-5)-(6x^2-5x-3)=-2x^2-x-8}`:

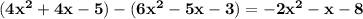Combine like terms and add -5 and 3 to get -2:

`=-2x^2+9x-2`

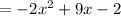
- So this is False.

e.)
`\bold{(3x^3-5+2x)-(5x-6+x^3)=2x^3-3x+1}`:

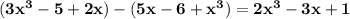Combine like terms and add -5 and 6 to get 1:

`=2x^3-3x+1`

- So this is True.

______________________________