Answer:
The percent error of the length of the soccer field is 1.4%
Explanation:
Actual length of soccer field = 500 feet
Length shown in measuring instrument = 493 feet
We need to find the percent error of the length of the soccer field.
The formula used to find percent error of the length of the soccer field is:
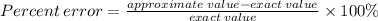
The exact length = 500
Approximate length = 493
Putting values and finding percent error
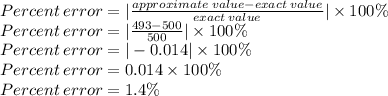
So, the percent error of the length of the soccer field is 1.4%