Answer:
6.9%.
Explanation:
From the given information it is clear that
Estimated distance from Mark's house to his school = 15.5 mi
Actual distance = 14.5 mi.
We need to find the percent error in Mark's estimate.

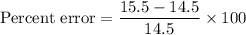
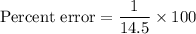


Round the percent to the nearest tenth.
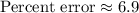
Therefore, the percent error is 6.9%.