Question

What is the solution of the equation?
4(y+2)=32
A 4
B 6
C -10
D 10

Answer 1

4(y+2)=32

Using the distributive property on the y and the 2, we get:

4y+8=32

Subtract the 8 on both sides and we get:
4y=24

Now, we can divide both sides by 4 and get the answer:
y=6

The answer is b

Answer 2

• 
  `\boxed{\sf{y=6}}`
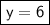
• The answer is B. 6.

Explanation:

Use a distributive property.

Isolate the term of y from one side of the equation.

• Distributive property:

`\Longrightarrow: \sf{A(B+C)=AB+AC}`

⇒: 4(y+2)=32

First, divide by 4 from both sides.

`\Longrightarrow:\sf{(4(y+2))/(4)=(32)/(4) }`

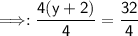
Solve.

⇒ 32/4=8

⇒ y+2=8

Subtract by 2 from both sides.

`\Longrightarrow \sf{y+2-2=8-2}`

Solve.

Subtract the numbers from left to right.

8-2=6

y=6

• Therefore, the correct answer is B. 6.

I hope this helps you! Let me know if my answer is wrong or not.