Question

A planet has an average distance to the sun of 0.66 AU.In two or more complete sentences explain how to calculate the orbital period of the planet and calculate it

Answer 1

T² = (4π²/GM)a³; where T is in Earth years, a is distance from sun in AU, M is the solar mass (1 for the sun), G is the gravitational constant.
In the given units, 4π²/G = 1
T² = 0.66³
T = 0.536 Earth years = 195.71 Earth days

Answer 2

Orbital period of the planet is 0.537 years.

Step-by-step explanation:

Given that,

A planet has an average distance to the sun of 0.66 AU,
`a=0.66\ AU=9.87* 10^(10)\ m`

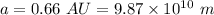
The orbital period of the planet is determined using Kepler's law of planetary motion. Mathematically, the Kepler's law is given by :

`T^2=(4\pi^2)/(GM)a^3`

`T^2=(4\pi^2)/(6.67* 10^(-11)* 1.98* 10^(30))* (9.87* 10^(10))^3`

`T=\sqrt{2.87* 10^(14)}\ s`

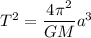
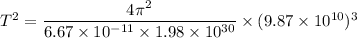
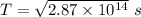
T = 16941074.34 s

or

T = 0.537 years

So, the orbital period of the planet is 0.537 years. Hence, this is the required solution.